Transfer functions of continuous-time systems

Problem 1: Consider the following system:

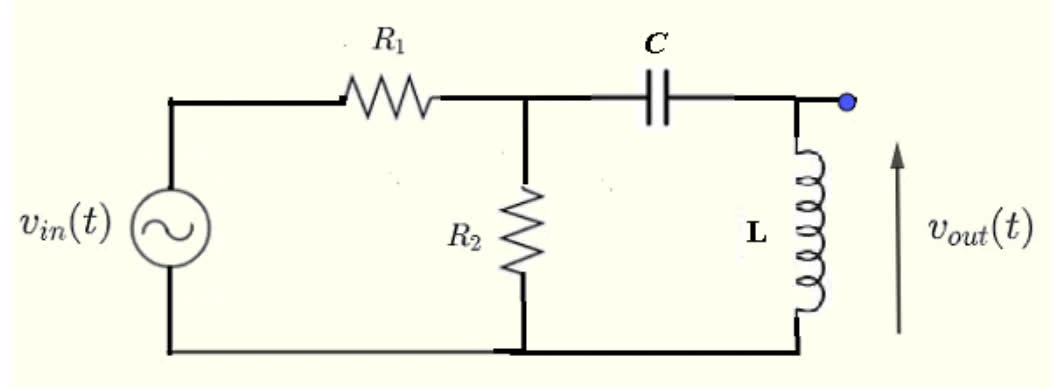assignment-1-circuit

Let $R_1 = 40\Omega, R_2 = 20\Omega, L = 10mH, C= 1\mu F$ . The input is $v_{in}$ the output is $v_{out}$ . Give both transfer function and state space representation for the system.

Solution

Given circuit is a second-order linear system due to presence of one inductor (L) and one capacitor (C).

Given transfer function $H(s)$ is given by the ratio over Laplace domain:

H(s) = \frac{V_{out}(s)}{V_{in}(s)}

Given that the impedance of the inductor $Z_l = sL$ and the impedance of the capacitor $Z_c = \frac{1}{sC}$ , the total impedance of the circuit is given by:

Z_{\text{total}} = \frac{1}{\frac{1}{sL} + sC}

Using voltage divider rule, the transfer function is given by:

H(s) = \frac{V_{out}(s)}{V_{in}(s)} = \frac{\frac{1}{sC}}{\frac{1}{sL} + \frac{1}{sC}}

Transfer functions of continuous-time systems

Étiquette

publié à

modifié à

durée

source

Vous pourriez aimer ce qui suit